Cameron Browne. Impossible fractals

It is generally possible to create a multibar illusion for most curves that can be drawn on the isometric grid. For example, Figure 11 shows the first three iterations of the Peano-Gosper curve or flowsnake [6] realised as an impossible multibar.

Figure 11. An impossible multibar Peano-Gosper curve.

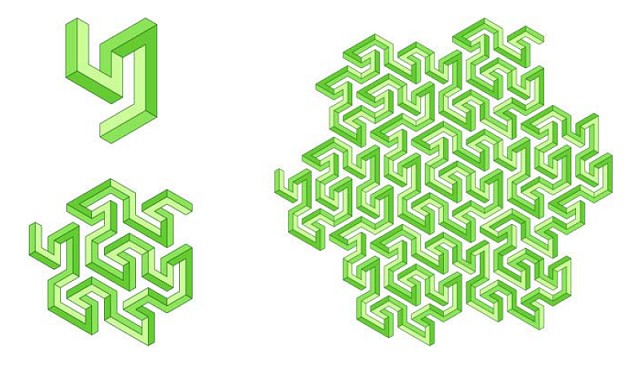
Similarly, Figure 12 (top left) shows Reutersvärd's "Meander" illusion [3] and its application to the first three iterations of the Hilbert curve, mapped to the isometric grid, to yield a Hilbert meander. This design is derived from the Devils's fork illusion and involves figure/background ambiguity. However, another illusion not found in the original Meander figure emerges, to yield two types of effect at once:

Figure 12. Reutersvärd's "Meander" and a Hilbert meander.

1. Figure/background ambiguity (as per the Devil's fork). Note that the original Meander figure is penetrated by the background from underneath, whereas the Hilbert meander is penetrated from all four sides without diminishing the effect.
2. Perspective ambiguity (as per a multibar). The top part of each Hilbert subfigure appears to run out of the page towards the left, while the bottom part appears to run out of the page towards the right. As the eye follows the curve around, each feature is interpreted and the mental map reoriented to the new perspective, and the previous perspective forgotten.

The Hilbert meander is best rendered as a black and white drawing as the Devil's fork and multibar colouring schemes are not compatible.