Cameron Browne. Impossible fractals

Figure 8 (left) shows an impossible multibar cube such as the one prominently featured in Escher's famous lithograph "Belvedere" [5]. Note that this figure violates the second multibar design rule as it contains adjacent same-coloured faces where the arms cross. This will be a problem with the multibar description of any non-planar shape, as the two overpassing colours and the two underpassing colours must be mutually exclusive; this figure really requires four colours.

Figure 8. An impossible multibar cube and the Menger sponge after one and three iterations.

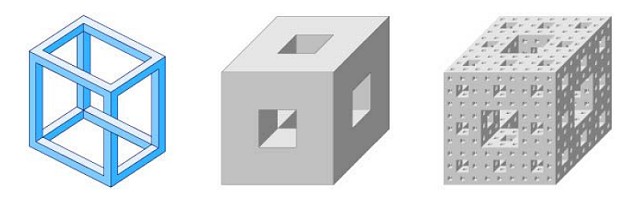
Figure 8 also shows the Menger sponge, which is the three-dimensional equivalent of the Cantor set, after one iteration (middle) and after three iterations (right). While the impossible multibar cube is topologically similar to the first iteration of the Menger sponge (except for the impossible crossing) it's difficult to apply the multibar technique to further iterations. However, the idea of cubic subdivision does yield another method for describing impossible objects used extensively by Reutersvärd [3]. This method is epitomised in his "Opus 2B" (Figure 9, top left) in which three overlapping cubes form a visual contradiction.

Figure 9. Subcube designs and Buch's "Cube in blue".

Figure 9 (bottom left) shows the tri-bar realised as a subcube design. This design is of particular interest as none of the overlapping subcubes form local contradictions (as they do in "Opus 2B") yet the overall figure is just as effective as the tri-bar. This design came before the tri-bar and was actually the first of Reutersvärd's many impossible figures, and is hence probably the first example of an impossible object designed specifically as an illusion. There exist examples of impossible figures in artwork dating back 1000 years, before the advent of classical perspective [3]. However, these generally appear to be "fixes" introduced by the artist to address compositional shortcomings (such as a pillar which would occlude an important foreground figure if drawn in correct perspective) rather than as deliberately ambiguous objects.

Figure 9 (middle, top) shows a square made of subcubes and Figure 9 (middle, bottom) shows a cube made of subcubes with local contradictions added. It can be seen that these figures contain a perceptual illusion but are not very elegant. The cubic subcube design in particular is confusing to the eye and becomes even more so if the subcubes are recursively subdivided into further subcubes. This approach is not amenable to fractal development.

Figure 9 (right) shows the key elements of Monika Buch's 1976 painting "Cube in blue" [3]. This is a clever realization of the impossible multibar cube as a subcube design, and exploits the fact that perpendicular arms share a common subcube of the same colour where they cross; it is ambiguous where the impossible multibar cube is contradictory. This design is also topologically similar to the first iteration of the Menger sponge and looks promising for recursive subdivision as a sponge-style fractal. Unfortunately, further subdivision again becomes overly confusing as the implied edges of the cubic frames at each level are lost - as is the perceptual effect - in the busy detail.

Figure 10. Ernst's "Nest of impossible cubes" and two variations.

The impossible is proving difficult to achieve in this case. However, Figure 10 shows a different style of perspective illusion, based on multiple planes, which may be recursively applied to a cube without affecting the illusion. Figure 10 (left) shows the basic design of Bruno Ernst's 1984 work "Nest of impossible cubes" - please note that Ernst's original [3] is more artfully executed both in its perspective and its texture. The first variation (middle) shows how the effect may be enhanced with curved arches, which allow a wider view of the interior portion of the wall. The second variation (right) shows that the effect can be achieved to some extent even without the arch.